How To Find Asymptote Of Log Function

Related Pages
Reciprocal Functions
Hyperbolas
More than lessons on Calculus

The post-obit diagram shows the different types of asymptotes: horizontal asymptotes, vertical asymptotes, and oblique asymptotes. Scroll down the page for more examples and solutions on how to discover asymptotes.

Asymptotes

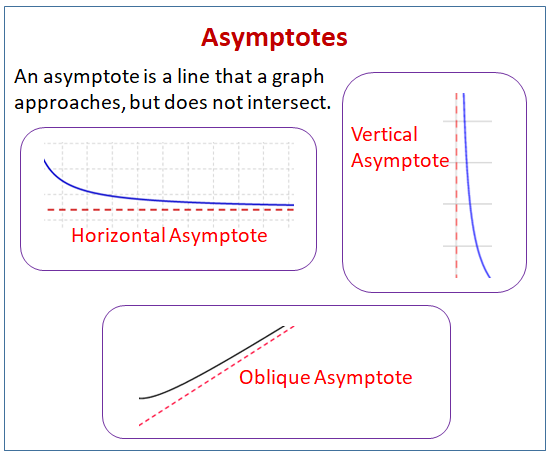
Vertical Asymptote

How to make up one's mind the Vertical Asymptote?

Method i: Utilise the Definition of Vertical Asymptote

The line x = a is called a Vertical Asymptote of the curve y = f(x) if at least 1 of the post-obit statements is truthful.

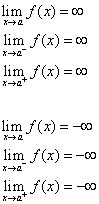
Method 2:

For rational functions, vertical asymptotes are vertical lines that correspond to the zeroes of the denominator.

Given the rational function, f(10)

Step 1: Write f(10) in reduced form

Step 2: if 10 – c is a cistron in the denominator then 10 = c is the vertical asymptote.

Example:
Find the vertical asymptotes of

Solution:
Method ane: Employ the definition of Vertical Asymptote.

If x is close to 3 only larger than 3, so the denominator ten – 3 is a pocket-sized positive number and 2x is close to viii. So, is a large positive number.

Intuitively, we see that

Similarly, if x is shut to 3 but smaller than 3, then x – iii is a pocket-size negative number and 2x is close to 8. So, is a big negative number.

The line ten = three is the vertical asymptote.

Method 2:

Footstep i: f(x) is already in reduced form.

Step 2: The denominator is 10 – 3, and then the Vertical Asymptote is at 10 = iii.

Finding Vertical Asymptotes of Rational Functions

What to look for, in order to notice vertical asymptotes of rational functions.

Evidence Video Lesson

Vertical Asymptotes of Rational Functions: Quick Fashion to Find Them

Example of finding vertical asymptotes of rational functions.

Show Video Lesson

Horizontal Asymptote

How to decide the horizontal Asymptote?

Method 1: Use the definition of Horizontal Asymptote

The line y = 50 is chosen a horizontal asymptote of the bend y = f(x) if either

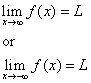
Method 2:

For the rational office, f(x)

If the degree of x in the numerator is less than the degree of x in the denominator then y = 0 is the horizontal asymptote.

If the caste of x in the numerator is equal to the degree of x in the denominator then y = c where c is obtained by dividing the leading coefficients.

Instance:
Find the horizontal and vertical asymptotes of the function.

Solution:
Method one:

Split both numerator and denominator by x.

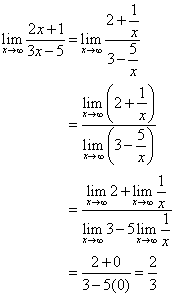
The line is the horizontal asymptote.

Method two:

The caste of x in the numerator is equal to the degree of 10 in the denominator.

Dividing the leading coefficients we get

The line is the horizontal asymptote.

Shortcut to Find Horizontal Asymptotes of Rational Functions

A couple of tricks that brand finding horizontal asymptotes of rational functions very easy to do The degree of a part is the highest power of x that appears in the polynomial. To find the horizontal asymptote, at that place are three easy cases.

If the degree of the numerator expression is less than the degree of the denominator expression, then the horizontal asymptote is y=0 (the x-centrality).
If the degree of the numerator is equal to the degree of the denominator, then you tin discover the horizontal asymptote past dividing the first, highest term of the numerator by the first, highest term of the denominator. This will simplify to y = some constant (just a number).
If the degree of the numerator is greater than the caste of the denominator, then there is no horizontal asymptote.

Bear witness Video Lesson

Oblique Asymptote or Slant Asymptote

Some curves have asymptotes that are oblique, that is, neither horizontal nor vertical.

If and so the line y = mx + b is chosen the oblique or camber asymptote because the vertical distances between the curve y = f(x) and the line y = mx + b approaches 0.

For rational functions, oblique asymptotes occur when the degree of the numerator is i more the degree of the denominator. In such a case the equation of the oblique asymptote tin can exist found by long partitioning.

Example:
Detect the asymptotes of the function

Solution:
Since the denominator xⁱⁱ + 1 is never 0, there is no vertical asymptote.

Since the degree of x in the numerator is greater than the degree of 10 in the denominator in that location is no horizontal asymptote.

Since the caste of x in the numerator is one greater than the degree of x in the denominator we can employ long partition to obtain the oblique asymptote.

And then, the line y = ten is the oblique asymptote.

Finding Slant Asymptotes of Rational Functions

This video describes when a rational role has a camber asymptote, briefly describe what a slant asymptote is, and and so do two examples.

Prove Video Lesson

Discover Asymptotes of a Rational Function (Vertical, Horizontal and Oblique/Slant)

This video shows how to observe the vertical asymptotes and a slant / oblique asymptotes of a rational function.

Show Video Lesson

Finding All Asymptotes of a Rational Function (Vertical, Horizontal, Oblique / Slant)

Here we await at a role and find the vertical asymptote and besides conclude that there are no horizontal asymptotes, only that an oblique asymptote does exist. Nosotros and so use long division to discover the oblique asymptote.

Show Video Lesson

Endeavour the gratuitous Mathway calculator and problem solver beneath to practice various math topics. Try the given examples, or type in your own problem and cheque your answer with the step-past-footstep explanations.
Mathway Calculator Widget